Greedy - Jump Game II

All diagrams presented herein are original creations, meticulously designed to enhance comprehension and recall. Crafting these aids required considerable effort, and I kindly request attribution if this content is reused elsewhere.

Difficulty : Easy

Greedy, 1D BST

Problem

You are given a 0-indexed array of integers nums of length n. You are initially positioned at nums[0].

Each element nums[i] represents the maximum length of a forward jump from index i. In other words, if you are at nums[i], you can jump to any nums[i + j] where:

• 0 <= j <= nums[i] and
• i + j < n

Return the minimum number of jumps to reach nums[n - 1]. The test cases are generated such that you can reach nums[n - 1].

Example 1:

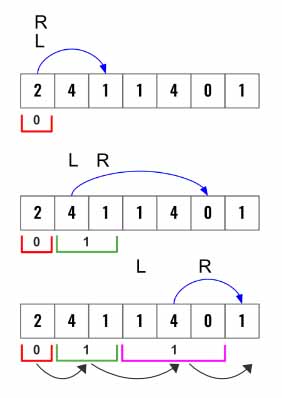
Input: nums = [2,3,1,1,4]
Output: 2
Explanation: The minimum number of jumps to reach the last index is 2. Jump 1 step from index 0 to 1, then 3 steps to the last index.

Example 2:

Input: nums = [2,3,0,1,4]
Output: 2

Solution

1. This is similar to 1D BST solution as need to find farthest index based on current left and right pointer.
2. Initially position left and right pointer to 0th index.
3. Then move right pointer based on how far it can reach.
4. Move the left pointer to 1+ current right pointer.
5. For each boundary increase jump count by 1.

In the beginning both left and right will point to 0 th index as the initial window. We will also set number of jumps needed to 0

left = right = 0
jumps = 0

Now we will have a while loop until the right pointer reaches end of the nums array.

while right < len(nums) -1:

We need to keep finding how farthest we could move in the current window. We would need a for loop for this.

  farthest = 0
  for i in range(left,right+1):
    farthest=max(farthest, i+nums[i])

Set left pointer to the left of the new window.

  left = right+1

Assign farthest to right.

  right = farthest

Increment jumps by 1.

  jumps+=1

Finally, return jumps.

return jumps

Final Code

Here is the full code.

def jumps(nums):
  left = right = 0 
  jumps = 0
  
  while right < len(nums) - 1:
    fasthest = 0
    for i in range(l, right+1):
      fasthest = max(fasthest, i + nums[i])
    
    left= right +1 
    right = fasthest
    jumps+=1
 return jumps